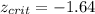Answer:
Null hypothesis:
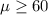
Alternative hypothesis:
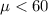
Since the sample size is large enough we can assume that the distribution for the statistic is normal so then in order to find the critical value for this test taking in count the significance level given of 0.05 we need to look in the normal standard distribution a value who accumulates 0.05 of the area in the left and would be:
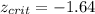
Explanation:
For this case we have the following data given:
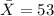 represent the sample mean
represent the sample mean
 represent the sample deviation
represent the sample deviation
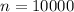 represent the sample size.
represent the sample size.
We want to test for this case if the true mean is less than 60 so then the system of hypothesis are:
Null hypothesis:
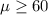
Alternative hypothesis:
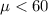
Since the sample size is large enough we can assume that the distribution for the statistic is normal so then in order to find the critical value for this test taking in count the significance level given of 0.05 we need to look in the normal standard distribution a value who accumulates 0.05 of the area in the left and would be: