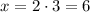Question

The digit in the tens placeof a two digit is three times that in the units place. if the digits are reversed , the new no will be 36 less than the original number .Find the original no.

pls help

Answer 1

If a number's ten digit is
`x` and the units digit is
`y`, then you can write the number as
`10x+y`.

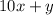Obviously, inverting the digits would lead to the number
`10y+x`.

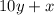Since this new number is 36 less than the original, we have

`10y+x = 10x+y-36 \iff 9x-9y=36\iff x-y=4`

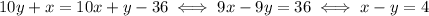
Since we also know that the digit in the tens place is three times that in the units place, we can add an equation to form a system:

`\begin{cases}x-y=4\\x=3y\end{cases}\iff 3y-y=4\iff y=2`

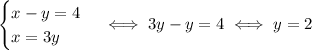
And thus
`x=2\cdot 3=6`