Question

A city council is considering funding a proposal to create a new city park. The council members will fund the proposal if they conclude that more than 60 percent of the city residents support the proposal. A survey of 2,000 randomly selected city residents will be conducted to investigate the level of support for the proposal. Let X represent the number of city residents in the sample who support the proposal. Assume that X is a binomial random variable.

Determine the mean and the standard deviation of the random variable X, assuming that 60 percent of city henudng proosal to create a new city park.

Answer 1

The mean of the the random variable X is 1200.

The standard deviation of the random variable X is 21.91.

Explanation:

The random variable X is defined as the number of city residents in the sample who support the proposal.

The random variable X follows a Binomial distribution with parameters n = 2000 and p = 0.60.

But the sample selected is too large and the probability of success is close to 0.50.

So a Normal approximation to binomial can be applied to approximate the distribution of X if the following conditions are satisfied:

1. np ≥ 10
2. n(1 - p) ≥ 10

Check the conditions as follows:

`np=2000* 0.60=1200>10\\\\n(1-p)=2000* (1-0.60)=800>10`

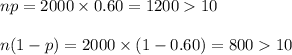
Thus, a Normal approximation to binomial can be applied.

So, the random variable X can be approximate by the Normal distribution .

Compute the mean of X as follows:

`\mu=np`

`=2000* 0.60\\=1200`

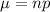
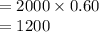
The mean of the the random variable X is 1200.

Compute the standard deviation of X as follows:

`\sigma=√(np(1-p))`

`=√(2000* 0.60* (1-0.60))\\=√(480)\\=21.9089\\\approx 21.91`

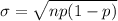
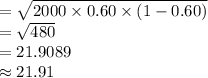
The standard deviation of the random variable X is 21.91.