Answer:
1)
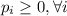
2)
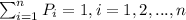
And for this case we have:
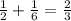
By the complement rule we can find the probability that the spinner land in a non black or red space:
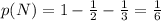
And then the probability distribution would be:
Color Red Black N
Prob. 1/3 1/2 1/6
Explanation:
For this case we have two possible outcomes for the spinner experiment:
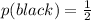
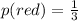
In order to have a probability distribution we need to satisfy two conditions:
1)
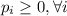
2)
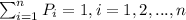
And for this case we have:
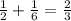
By the complement rule we can find the probability that the spinner land in a non black or red space:
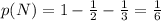
And then the probability distribution would be:
Color Red Black N
Prob. 1/3 1/2 1/6