Question

A 0.1 significance level is used for a hypothesis test of the claim that when parents use a particular method of gender​ selection, the proportion of baby girls is greater than 0.5. Assume that sample data consists of 78 girls in 144 ​births, so the sample statistic of StartFraction 13 Over 24 EndFraction results in a z score that is 1 standard deviation above 0. Complete parts​ (a) through​ (h) below.

(a) Identify the null hypothesis and the alternative hypothesis. Choose the correct answer below.
a. H0:p=0.5 H1:P≠0.5.
b. H0:p≠0.5 H1:p=0.5.
c. H0:p=0.5 H1:p>0.5.
d. H0:p=0.5 H1:p<0.5.
(b) What is the value of α? (Type an integer or a decimal.)
(c) What is the sampling distribution of the sample statistic?
a. Normal distribution.
b. χ2.
c. Student (t) distribution.
(d) Is the test two-tailed, left-tailed, or right-tailed?
a. Right-tailed.
b. Left-tailed.
c. Two-tailed.
(e) What is the value of the test statistic? (Type an integer or a decimal).
(f) What is the P-value? (Round to four decimal places as needed.)
(g) What are the critical values(s)? (Round to three decimal places as needed. Use a comma to separate answers as needed.)
(h) What is the area of the critical region? (Round to two decimal places as needed.)

Answer 1

(a) Null Hypothesis,
`H_0` : p = 0.50

Alternate Hypothesis,
`H_A` : p > 0.50

(b) The value of level of significance (α) given in the question is 0.10.

(c) The sampling distribution of the sample statistic is Normal distribution.

(d) This test is right-tailed.

(e) The value of z test statistics is 0.96.

(f) The P-value is 0.1685.

(g) At 0.10 significance level the z table gives critical value of 1.282 for right-tailed test.

(h) We conclude that the proportion of baby girls is equal to 0.50.

Explanation:

We are given that a 0.1 significance level is used for a hypothesis test of the claim that when parents use a particular method of gender​ selection, the proportion of baby girls is greater than 0.5.

Assume that sample data consists of 78 girls in 144 ​births.

Let p = population proportion of baby girls

(a) So, Null Hypothesis,
`H_0` : p = 0.50 {means that the proportion of baby girls is equal to 0.50}

Alternate Hypothesis,
`H_A` : p > 0.50 {means that the proportion of baby girls is greater than 0.50}

(b) The value of level of significance (α) given in the question is 0.10.

(c) The sampling distribution of the sample statistic is Normal distribution.

(d) This test is right-tailed as in the alternative hypothesis we are concerned for proportion of baby girls that is greater than 0.50.

(e) The test statistics that would be used here One-sample z test for proportions;

T.S. =
`\frac{\hat p-p}{\sqrt{(\hat p(1-\hat p))/(n) } }` ~ N(0,1)

where,
`\hat p` = sample proportion of baby girls =
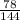`(78)/(144)` = 0.54

n = sample of births = 144

So, the test statistics =
`\frac{0.54-0.50}{\sqrt{(0.54(1-0.54))/(144) } }`

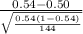
= 0.96

The value of z test statistics is 0.96.

(f) The P-value of the test statistics is given by;

P-value = P(Z > 0.96) = 1 - P(Z < 0.96)

= 1 - 0.8315 = 0.1685

(g) Now, at 0.10 significance level the z table gives critical value of 1.282 for right-tailed test.

(h) Since our test statistic is less than the critical value of z as 0.96 < 1.282, so we have insufficient evidence to reject our null hypothesis as it will not fall in the rejection region (which was to the right of value of 1.282) due to which we fail to reject our null hypothesis.

Therefore, we conclude that the proportion of baby girls is equal to 0.50.