Answer:

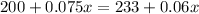
We can subtract from both sides 0.06x and we got:
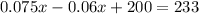
And now we can subtract 200 from both sides we got:
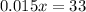
And dividing both sides by 0.015 we got:
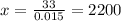
And then the final anwer would be x =2200
Explanation:
For this case we have the following function for the total sales of Lucy is given by:
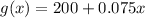
And the function for the total sales of Zachary is given by:
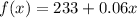
And we want to determine the value of x, in dollars, that will make their weekly pay the same, so we can set equal the two functions:

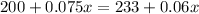
We can subtract from both sides 0.06x and we got:
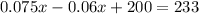
And now we can subtract 200 from both sides we got:
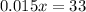
And dividing both sides by 0.015 we got:
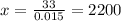
And then the final anwer would be x =2200