Answer:
Option B.
Explanation:
Note: Given roots are not in proper format.
Consider the polynomial function f(x) has roots 0, 3, and
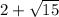 .
.
According to irrational root theorem if
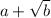 is root of a polynomial P(x), then
is root of a polynomial P(x), then
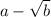 is also a root of polynomial P(x).
is also a root of polynomial P(x).
Since
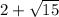 is an irrational root of the function f(x). By irrational root theorem
is an irrational root of the function f(x). By irrational root theorem
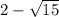 is also a root of function f(x).
is also a root of function f(x).
Therefore, the correct option is B.