We have been given that in ΔRST, s = 93 inches, ∠S=123° and ∠T=28°. We are asked to find the length of r to the nearest 10th of an inch.
We will use law of sines to solve for side r.
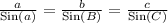 , where a, b and c are corresponding sides to angles A, B and C respectively.
, where a, b and c are corresponding sides to angles A, B and C respectively.
Let us find measure of angle S using angle sum property of triangles.
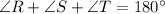
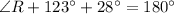
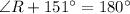

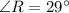
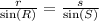
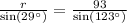
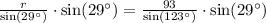
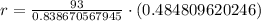
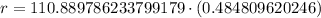

Upon rounding to nearest tenth, we will get:
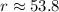
Therefore, the length of r is approximately 53.8 inches.