Question

I don’t understand how to do this problem. How would I solve it?

Answer 1

About 2.569 seconds

Explanation:

To solve this problem, you can separate it into two half parabolas. The beginning part is initial upward rising. To calculate the amount of time that this part of the dive takes, you can use the formula
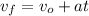`v_f=v_o+at`, where
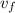`v_f` is the final velocity,
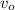`v_o` is the initial velocity, a is the acceleration due to gravity, and t is the amount of time it takes. You know that the final velocity is 0, since the diver is reaching the terminal of their dive. Therefore, you can set up the following equation (assuming that the acceleration due to gravity is 32ft/s^2):

`0=5+(-32)t`

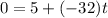
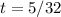`t=5/32` seconds

To find the height that this indicates the diver has risen, you can plug this time into the following formula:
`d=v_o t+(1)/(2)at^2`

`d=5(5/32)+(1)/(2)\cdot 32\cdot (5/32)^2\approx 1.172` feet

For the second part, you can use the equation
`d=v_o t+(1)/(2)at^2` again. Since the initial velocity for this part is 0, you can set up the following equation:

`92+1.172=(1)/(2)\cdot 32 \cdot t^2`

`t \approx 2.413`

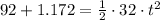
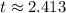
Adding this to the time that the first part of the dive takes, you get a total of about 2.569 seconds. Hope this helps!