Question

Tristan purchased a new car in 2000 for $22,800. The value of the car has been depreciating exponentially at a constant rate. If the value of the car was $10,400 in the year 2006, then what would be the predicted value of the car in the year 2016, to the nearest dollar?

Answer 1

Cost of the car in year 2016 will be $2811.

Explanation:

Cost price of the car = $22800

Value of the car has been depreciating exponentially, so the formula for value of the car after t years,

`P(t)=A(1-(r)/(100))^(t)`

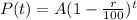
Here P(t) = Final value after t years

A = Cost price or initial value

r = rate of depreciation

t = duration in years

`P(6)=22800(1-(r)/(100))^(6)`

`10400=22800(1-(r)/(100))^(6)`

`(10400)/(22800)=(1-(r)/(100))^(6)`

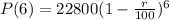
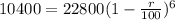
0.87737 =
`(1-(r)/(100))`

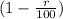
r = 100(1 - 0.87737)

r = 12.26%

Now cost of the car in year 2016 (After 16 years)

P(16) =
`22800(1-(12.26)/(100))^(16)`

=
`22800(0.87737)^(16)`

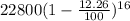
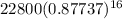
= 2810.99

= $2811

Therefore, cost of the car in year 2016 will be $2811.