Question

An ice skater spins at 2.5 rev/s when his arms are extended. He draws his arms in and spins at 10.0 rev/s. By what factor does his moment of inertia change in the process?

Answer 1

The moment of inertia decreased by a factor of 4

Step-by-step explanation:

Given;

initial angular velocity of the ice skater, ω₁ = 2.5 rev/s

final angular velocity of the ice skater, ω₂ = 10.0 rev/s

During this process we assume that angular momentum is conserved;

I₁ω₁ = I₂ω₂

Where;

I₁ is the initial moment of inertia

I₂ is the final moment of inertia

`I_2 = (I_1 \omega_1)/(\omega_2) = (I_1*2.5)/(10) \\\\I_2 = 0.25I_1 = (1)/(4)I_1`

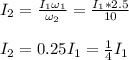
Therefore, the moment of inertia decreased by a factor of 4