Question

If 3x and 71/x are two prime numbers V x equivalent to R, then number of x so that 3x + 71/x = 10 is/ are

Answer 1

x = 5/3

Explanation:

Guven two prime numbers to be 31/x and 71/x, if their sum is 10 as given;

3x + 71/x = 10 then to find the value of x, the following steps must be taken;

Step 1

Find the LCM of the given equation;

3x + 71/x = 10

`(3x^(2)+71 )/(x) =10\\`

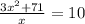
Step 2:

Cross multiplying;

`3x^(2) +71=10x\\3x^(2) -10x+71 =0\\`

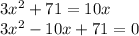
Using the general formula to get the value of x;

x = -b±√b²-4ac/2a

a=3, b=-10, c=71

= 10±√(-10)²-4(3)(71)/2(3)

= 10±√100-852/6

= 10±√-752/6

= 10±27.4i/6

= 10+27.4i/6 or 10-27.4i/6

x = 5/3+27.4i/6 or 5/3-27.4i/6

Since the values of x are real values then, our answer will be the real part of the complex number gotten.

x = 5/3