Answer:
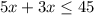 represents all possible combinations of x tulips and y carnations
represents all possible combinations of x tulips and y carnations
Explanation:
We are supposed to find inequality represents all possible combinations of x tulips and y carnations
Cost of 1 tulip = $5
Cost of x tulips = 5x
Cost of 1 carnation =$3
Cost of y carnations = 3y
We are given that she does not want to spend more than $45.
So, She can spend less than or equal to 45
So, required inequality :
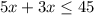
So, Option B is true
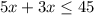 represents all possible combinations of x tulips and y carnations
represents all possible combinations of x tulips and y carnations