Question

Which function is the result of vertically shrinking ƒ(x) = (x + 1)2 by a factor of 1∕6?

Question 6 options:

A) ƒ(x) = (6x + 1)2

B) ƒ(x) = (1∕6x + 1)2

C) ƒ(x) = 6(x + 1)2

D) ƒ(x) = 1∕6 (x + 1)2

Answer 1

ƒ(x) = (1∕6x + 1)^2

Explanation:

Answer 2

`y=(1)/(6)(x+1)^2` is the result of vertically shrinking

Explanation:

Vertical stretch or shrink

`y=c \cdot f(x)`

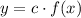
a stretch by a factor of c if c>1

a shrink by a factor of c if c<1

c is the factor

We are given that factor = 1/6 <1

So, Vertical shrink

So,
`y = (1)/(6) \cdot f(x)`

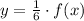
We are given that
`f(x) = (x + 1)^2`

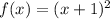
So,
`y=(1)/(6)(x+1)^2`

Option D is true

Hence
`y=(1)/(6)(x+1)^2` is the result of vertically shrinking