Question

If x= -1 and y=2 is the solution of the simultaneous equations,

ax-by= 1
ay+bx= - 7
find the value of a and b with the proper steps

Answer 1

a = -3, b = 1

Explanation:

1. Plug in the given values into the equations:

a(-1) - b(2) = 1
a(2) + b(-1) = -7

2. Isolate a in the first equation:

a(-1) - b(2) = 1 = a · -1 - b · 2 = 1

-a - 2b = 1

-a -2b+2b = 1+2b

-a = 1 + 2b

`(-a)/(-1) =(1)/(-1) +(2b)/(-1)`

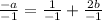
a = -1 - 2b

3. Substitute "a = -1 - 2b" in for a in the second equation:

(-1 - 2b)2 + b(-1) = -7

4. Simplify:

-2 - 4b + b(-1) = -7

-2 - 4b -b = -7

-2 -5b = -7

5. Isolate for b:

-2+2 - 5b = -7+2

-5b = -5

`(-5b)/(-5) =(-5)/(-5)`

b = 1

6. Substitute the b value into an equation to solve for a:

a = -1 -2 · 1

a = -3 · 1

a = -3

hope this helps!