Answer:
The value of z test statistics is 0.77.
Explanation:
We are given that in a national survey, middle school students report spending 3.1 hours per week on homework.
Mr. Jones asks his class of 24 students about the amount of time they spend on homework, and the average for his class is 3.4 hours per week. Mr. Jones assumes the standard deviation of homework time in the population is 1.9 hours per week.
Let
 = true average time students spend on homework.
= true average time students spend on homework.
So, Null Hypothesis,
 :
:
 = 3.1 hours/week {means that Mr. Jones students study equal to the national average}
= 3.1 hours/week {means that Mr. Jones students study equal to the national average}
Alternate Hypothesis,
 :
:
 > 3.1 hours/week {means that Mr. Jones students study more than the national average}
> 3.1 hours/week {means that Mr. Jones students study more than the national average}
The test statistics that would be used here One-sample z test statistics as we know about the population standard deviation;
T.S. =
 ~ N(0,1)
~ N(0,1)
where,
 = sample mean time they spend on homework = 3.4 hours/week
= sample mean time they spend on homework = 3.4 hours/week
 = population standard deviation = 1.9 hours/week
= population standard deviation = 1.9 hours/week
n = sample of students = 24
So, the test statistics =
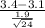
= 0.77
Hence, the value of z test statistics is 0.77.