Answer:
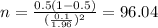
Then the minimum sample size in order to satisfy the condition of 0.1 for the margin of error is 97 and since the sample used is n =100 we can conclude that is sufficient and the best answer would be:
D. Yes.
Explanation:
In order to find the critical value we need to take in count that we are finding the interval for a proportion, so on this case we need to use the z distribution. We know that we require a 95% of confidence, our significance level would be given by
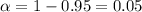 and
and
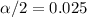 . And the critical value would be given by:
. And the critical value would be given by:
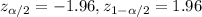
The margin of error for the proportion interval is given by this:
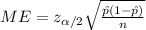 (a)
(a)
We want a margin of error of
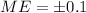 and we are interested in order to find the value of n, if we solve n from equation (a) we got:
and we are interested in order to find the value of n, if we solve n from equation (a) we got:
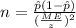 (b)
(b)
Since we don't have prior info for the population proportion we can use as estimator the value of 0.5. And replacing into equation (b) the values from part a we got:
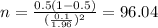
Then the minimum sample size in order to satisfy the condition of 0.1 for the margin of error is 97 and since the sample used is n =100 we can conclude that is sufficient and the best answer would be:
D. Yes.