Question

A particular brand of tires claims that its deluxe tire averages at least 50,000 miles before it needs to be replaced. From past studies of this tire, the standard deviation is known to be 8,000. A survey of owners of that tire design is conducted. Of the 28 tires surveyed, the mean lifespan was 46,300 miles with a standard deviation of 9,800 miles. Using alpha = 0.05, is the data highly consistent with the claim? Note: If you are using a Student's t-distribution for the problem, you may assume that the underlying population is normally distributed. (In general, you must first prove that assumption, though.)

Answer 1

`z=(46300-50000)/((9800)/(√(28)))=-1.998`

Now we can calculate the p value using the alternative hypothesis with this probability:

`p_v =P(z<-1.998)=0.0229`

The p value for this case is significantly lower than the value of
`\alpha=0.05` so then we can reject the null hypothesis at this signficance level and we have enough evidence to conclude that the true mean for the deluxe tire is less than 50000 and the claim is not correct.

Explanation:

Information given

`\bar X=46300` represent the sample mean for the lifespans

`\sigma=9800` represent the population standard deviation

`n=28` sample size

`\mu_o =50000` represent the value to verify

`\alpha=0.05` represent the significance level

z would represent the statistic

`p_v` represent the p value

System of hypothesis

The idea for this case is verify if the deluxe tire averages at least 50,000, so then the system of hypothesis are:

Null hypothesis:
`\mu \geq 50000`

Alternative hypothesis:
`\mu < 50000`

We know the population deviation so then the correct test stattistic would be:

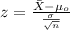`z=(\bar X-\mu_o)/((\sigma)/(√(n)))` (1)

Replacing the info given we got:

`z=(46300-50000)/((9800)/(√(28)))=-1.998`

Now we can calculate the p value using the alternative hypothesis with this probability:

`p_v =P(z<-1.998)=0.0229`

The p value for this case is significantly lower than the value of
`\alpha=0.05` so then we can reject the null hypothesis at this signficance level and we have enough evidence to conclude that the true mean for the deluxe tire is less than 50000 and the claim is not correct.