Question

Consider a disk, having mass and radius , that spins rapidly about an axle at its center, spinning with an angular velocity . The disk's axle is connected by a thin, massless rod of length to a central pivot. The end of the massless rod is fixed in place at that pivot, but it can freely rotate in all directions. 1) If the disk's mass and radius were each doubled, while keeping the other quantities (, , and ) fixed, by what factor would the rate of precession change? I.e., what is ?

Answer 1

1/2

Step-by-step explanation:

Solution:-

- The torque ( T ) induced at the pivotal point of the massless rod-disk assembly is given by:

T = M*g*r*sin ( θ )

Where,

M: The mass of the disk

r: the radius of the disk

θ: The precession angle

- The rate of change in angular momentum of the disk ( dL / dt ) is due to the induced torque ( T ) given by:

dL / dt = M*g*r*sin ( θ )

Hence,

dL = M*g*r*sin ( θ ). dt ... Eq1

- The change in precession angle ( dθ ) is a orthogonal component of change in angular momentum ( dL ) :

dθ = dL / ( L*sin ( θ ) ) ... Eq2

- Combine Eq1 and Eq2:

dθ = M*g*r*sin ( θ ). dt / ( L*sin ( θ ) )

dθ/dt = M*g*r / L ... Eq3

Where,

- The angular momentum of the disk is given by the product of moment of inertia ( I ) and angular velocity ( w ):

`L = I*w\\\\L = (1)/(2)*M*r^2*w`

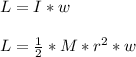
- Substitute the relationship of angular momentum of disk ( L ) into Eq3:

dθ/dt = M*g*r / 0.5*M*r^2*w

- Hence, the rate of change of precession ( dθ/dt ) is given by:

dθ/dt = 2*g / r*w

- If we double the mass ( M to 2M ) there is no effect on the rate of change of precession ( dθ/dt ). However, if we double the radius of the disk the rate of precession ( dθ/dt ) is halved due to the inverse relationship between the rate of precession ( dθ/dt ) and radius of the disk.

Answer:The factor of change would be ( 1 / 2 ) of the initial rate of change in precession.