Answer:
66.94N
Step-by-step explanation:
To find the force you use the second Newton law, which is given by:
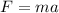 (1)
(1)
m: mass of the ball 5.85g
the acceleration is:
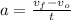
vf: final velocity = 128mph = 57.21m/s
vo : initial velocity = 0m/s
t: time = 5ms =5*10^{-3}s
by computing the acceleration and replacing it in the equation (1) you obtain:
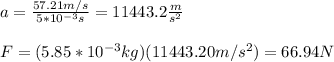
hence, the force applied by Serena on the ball is 66.94N