Answer:
Every day, the mass of the sunfish is multiplied by a factor of [ln(1.34)/6].
Explanation:
You have the following function:
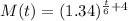
To know what is the factor that multiplies the mass of the sunfish each day, you derivative the function M(t):
![(dM(t))/(dt)=(1.34)^{(t)/(6)+4}((1)/(6))ln(1.34)\\\\(dM(t))/(dt)=(ln(1.34))/(6)[(1.43)^{(t)/(6)+4}]\\\\(dM(t))/(dt)=(ln(1.34))/(6)M(t)](https://img.qammunity.org/2021/formulas/mathematics/college/6w086hb34nrdhczugndz8mlak3z4myw52q.png) (1)
(1)
where you have used the following general derivative:
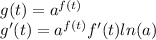
The derivative give you the increase in the mass per day (because t is days). By the expression (1) you can conclude that each day the mass increase a factor of [ln(1.34)/6].
Every day, the mass of the sunfish is multiplied by a factor of [ln(1.34)/6].