Question

zach wants to factor 3x^5-81x^2. He knows that the difference of cubes formula is a^3-b^3=(a-b)(a^2+ab+b^2) zach decides to use the formula. first, he factors 3x^2 out of the expression.next, he identifies a and b. finally, he factors the expression according to the formula. his work is shown here

Answer 1

3x²(x - 3)(x² + 3x + 9)

Explanation:

Given

3
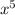`x^(5)` - 81x² ← factor out 3x² from each term

= 3x²(x³ - 27)

x³ - 27 ← is a difference of cubes and factors in general as

a³ - b³ = (a - b)(a² + ab + b²)

Thus

x³ - 27

= x³ - 3³ → with a = x and b = 3

= (x - 3)(x² +3x + 9)

Hence

3
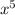`x^(5)` - 81x² = 3x²(x - 3)(x² + 3x + 9) ← in factored form