Answer:
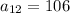
Explanation:
Arithmetic sequence
General form of an arithmetic sequence:
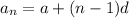
where:
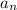 is the nth term
is the nth term- a is the first term
- d is the common difference between terms
Given:
To find the common difference (d), substitute the given values into the general formula and solve:
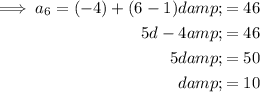
Therefore, the equation for the nth term is:
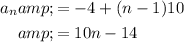
To find the 12th term, substitute n = 12 into the equation:
