Question

To the nearest cubic inch, what is the volume of the
right triangular prism?

Answer 1

The Volume of right triangular prism is 240 cubic inches

Explanation:

Volume of right triangular prism =
`\text{Area of base} * height`

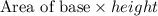
In triangle ABC

AC = Hypotenuse = 13 in.

AB = Height

BC = Base = 12 in.

Using Pythagoras theorem

`Hypotenuse^2=Perpendicular^2+base^2\\13^2=Perpendicular^2+12^2\\√(13^2-12^2)=Perpendicular`

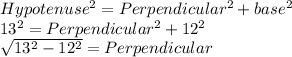
5=Perpendicular

Area of base =
`(1)/(2) * Base * Height = (1)/(2) * 12 * 5 = 30 in^2`

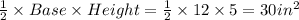
Height of prism = 8 in

Volume of right triangular prism =
`\text{Area of base} * height`

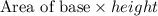
Volume of right triangular prism =
`30 * 8 = 240 in^3`

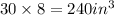
Hence The Volume of right triangular prism is 240 cubic inches