Question

Equation of circle: mastery test HELPPPP PLS !!

Answer 1

PQ is the diameter so the midpoint of PQ is the center of the circle. if we suppose the center point of the circle called o then ;

`o = (pq)/(2) \\`

`pq = \sqrt{ { (- 10 - 6})^(2) + ({10 - ( - 2)})^(2) }`

`o = (( -10 ,10) + (6 , - 2) )/(2) \\`

`o = (( - 10 +6 ,10 - 2))/(2) \\`

`o = ( ( - 4)/(2) , (8)/(2) ) \\`

`o = ( - 2,4)`

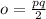
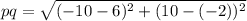
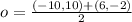
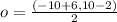
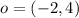
For the second step we need to find the radius of the circle which has the half the PQ distance measure, so we have to find the the PQ distance and divide it by 2 :

_____________________________________________

As u know the distance between 2 point is going to find by following formula :

`pq = \sqrt{ {(x(p) - x(q)})^(2) + {(y(p) - y(q)})^(2) } \\`

`pq = \sqrt{ ({ - 10 - 6})^(2) + ({10 - ( - 2)})^(2) }`

`pq = \sqrt{ ({ - 16})^(2) + ({12})^(2) }`

`pq = √(256 + 144)`

`pq = √(400)`

`pq = 20`

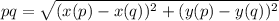
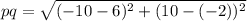
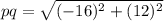
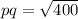
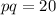
Thus :

`radius = (pq)/(2) \\`

`radius = (20)/(2) \\`

`radius = 10`

_____________________________________________

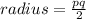
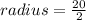
Ok we're almost done cuz we have what we needed:

If we suppose that the first coordinate of the center is a and the second coordinate of the center is b and the radius shown by r then :

`({x -a})^(2) + ( {y - b})^(2) = {r}^(2)`

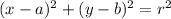
Is the equation of the circle in the standar form thus :

`({x - ( - 2)})^(2) + ({y - 4})^(2) = {10}^(2) \\`

`({x + 2})^(2) + ({y - 4})^(2) = 100`

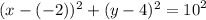
As u can see option B is the correct answer.

And we're done ...

Have a great day ♡♡♡♡♡