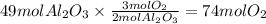Question

What mass of aluminum is produced by the decomposition of 5.0 kg? How many moles of oxygen are produced?

Answer 1

2.6 × 10³ g Al

74 mol O₂

Step-by-step explanation:

Aluminum oxide decomposes into aluminum and oxygen, according to the following balanced equation.

2 Al₂O₃ ⇒ 4 Al + 3 O₂

Step 1: Calculate the moles corresponding to 5.0 kg of aluminum oxide

The molar mass of aluminum oxide is 101.96 g/mol.

`5.0 * 10^(3) g * (1mol)/(101.96g) = 49mol`

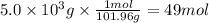
Step 2: Calculate the mass of aluminum formed

We will use the following relations.

• The molar ratio of Al₂O₃ to Al is 2:4

• The molar mass of Al is 26.98 g/mol

`49molAl_2O_3 * (4molAl)/(2molAl_2O_3) * (26.98gAl)/(1molAl) = 2.6 * 10^(3) gAl`

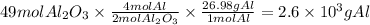
Step 3: Calculate the moles of oxygen produced

The molar ratio of Al₂O₃ to O₂ is 2:3. Then,

`49molAl_2O_3 * (3molO_2)/(2molAl_2O_3) =74molO_2`