Answer:
C. x = 6; straight vertical line
Explanation:
you have the following equation in polar coordinates:
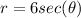
To find the equation in rectangular coordinates you take into account the following transformations:

Next, you replace and obtain:
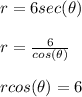
but, you have that x = rcos(θ), hence, x = 6
C. x = 6; straight vertical line