Question

Which is the equation of a hyperbola centered at the origin with vertex (2, 0) that passes through

4,3 )?

Answer 1

B

Explanation:

edge

Answer 2

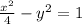`(x^(2))/(4) - y^(2) = 1` (Option B)

Explanation:

The hyperbola has the following vertices:
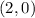`(2,0)` and
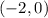`(-2,0)`. The first constant of the hyperbola is:

`2\cdot a = 4`

`a = 2`

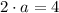
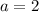
The other constant is determined by using the standard equation of the hyperbola and replacing all known variables:

`(4^(2))/(4) - (3)/(b^(2)) = 1`

`4 - (3)/(b^(2)) = 1`

`(3)/(b^(2)) = 3`

`b^(2) = 1`

`b = 1`

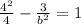
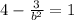
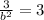
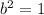
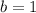
The equation of the hyperbola in standard form is:

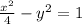`(x^(2))/(4) - y^(2) = 1` (Option B)