Answer:
The vertices are (±1/2, 0), the asymptote is y = -6x, and the foci of the hyperbola is
How to determine the vertices, asymptotes, and the foci?
The equation of the hyperbola is given as:
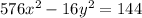
Divide through by 144
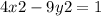
Rewrite as:
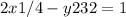
Hence, the vertices are (±1/2, 0), the asymptote is y = -6x, and the foci of the hyperbola is ⁽₍₋₋₂₋₋ ₃₇ ° 0₎⁾ Hope This helps Have A Nice Day!