Question

Consider a drug testing company that provides a test for marijuana usage. Among 310 tested​ subjects, results from 28 subjects were wrong​ (either a false positive or a false​ negative). Use a 0.05 significance level to test the claim that less than 10 percent of the test results are wrong.

Answer 1

`z=\frac{0.0903 -0.1}{\sqrt{(0.1(1-0.1))/(310)}}=-0.569`

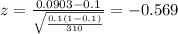
The p value for this case can be calculated with this probability:

`p_v =P(z<-0.569)=0.285`

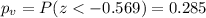
Since the p value is higher than significance level we don't have enough evidence to conclude that the true proportion is significantly less than 0.1

Explanation:

Information given

n=310 represent th sample selected

X=28 represent the subjects wrong

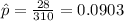`\hat p=(28)/(310)=0.0903` estimated proportion of subjects wrong

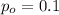`p_o=0.1` is the value to verify

`\alpha=0.05` represent the significance level

t would represent the statistic

`p_v` represent the p value

System of hypothesis

We want to test the claim that less than 10 percent of the test results are wrong ,and the hypothesis are:

Null hypothesis:
`p\geq 0.1`

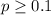
Alternative hypothesis:
`p < 0.1`

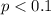
The statistic is given by:

`z=\frac{\hat p -p_o}{\sqrt{(p_o (1-p_o))/(n)}}` (1)

Replacing the info we got:

`z=\frac{0.0903 -0.1}{\sqrt{(0.1(1-0.1))/(310)}}=-0.569`

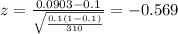
The p value for this case can be calculated with this probability:

`p_v =P(z<-0.569)=0.285`

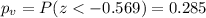
Since the p value is higher than significance level we don't have enough evidence to conclude that the true proportion is significantly less than 0.1