Question

What’s the correct answer for this?

Answer 1

12.72

Explanation:

To find the length of PQ, use the sine ratio. This is because you need one that includes the hypotenuse and the side opposite the given angle and sine:

`sineX=(opposite)/(hypotenuse)`

Insert the values, and let x be the unknown value of PQ:

`sin58=(x)/(15)`

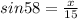
Solve for x. Multiply both sides by 15 and simplify:

`15*(sin58)=15*((x)/(15))\\\\15*sin58=x`

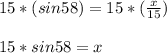
Insert into a calculator:

`x=15*sin58\\\\x=12.720`

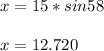
Round to the nearest hundredth (two decimal places):

`x=12.72`

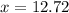
PQ is 12.72 units long.

:Done

Answer 2

12.72 = PQ

Explanation:

This is a right triangle, so we can use trig functions

sin theta = opp/ hyp

sin 58 = PQ / QR

sin 58 = PQ / 15

Multiply each side by 15

15 sin 58 = PQ

15 ( .848) = PQ

12.72 = PQ