Answer:
After 7.1 years
Explanation:
The compound interest formula is given by:
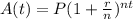
Where A(t) is the amount of money after t years, P is the principal(the initial sum of money), r is the interest rate(as a decimal value), n is the number of times that interest is compounded per unit year and t is the time in years for which the money is invested or borrowed.
In this question:
We have to find t, for which
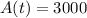 when
when
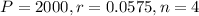
So
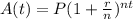

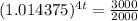
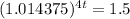
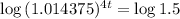
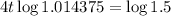
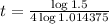
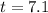
After 7.1 years