Question

Show that the set a = (1, 2, 1); b = (0, 1, 0); c = (-2, 0, 2) is linearly dependent.​

Answer 1

By definition of linear independence, the vectors in the set {a, b, c} are independent if

`k_1\vec a+k_2\vec b+k_3\vec c=\vec0`

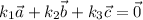
can only be obtained with the choice of k₁ = k₂ = k₃ = 0.

This vector equation corresponds to the system of linear equations

`\begin{cases}k_1 - 2k_3 = 0 \\ 2k_1 + k_2 = 0 \\ k_1 + 2k_3 = 0\end{cases}`

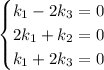
The first equation says k₁ = 2 k₃, while the third one says k₁ = -2 k₃. This can only be possible if k₁ = k₃ = 0, and from the second equation it follows that k₂ = 0. So the given set is linearly independent (and *not* dependent).