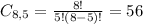Question

Each of 8 students wishes to buy a particular textbook, but only 5 textbooks are available. How could one express the number of ways those textbooks could be distributed among the students?

Answer 1

The number of ways those textbooks could be distributed among the students is expressed by a combinations of 8(books) to 5(students), which is
`C_(8,5) = (8!)/(5!(8-5)!) = 56`

Explanation:

The order in which the students get the textbooks is not important. For example, A,B,C,D and E getting the textbooks is the same outcome as B,A,C,D and E. So we use the combinations formula to solve this question.

Combinations formula:

`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

In this problem

5 textbooks to 8 students. So

`C_(8,5) = (8!)/(5!(8-5)!) = 56`

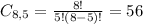
The number of ways those textbooks could be distributed among the students is expressed by a combinations of 8(books) to 5(students), which is
`C_(8,5) = (8!)/(5!(8-5)!) = 56`