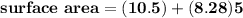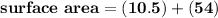Answer:

Step-by-step explanation:
The missing diagram is attached in the file below:
The formula for calculating the surface of triangular prism is expressed as:

where ;
base b = 28 mm = 2.8 cm
height h of the triangle = 3.75 cm
the sides of the triangle
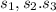 = 2.8 , 4 and 4 respectively
= 2.8 , 4 and 4 respectively
the height if the prism H = 5 cm