Answer:
The probability that in a random sample of 100 CSU graduates the error is within 5% of the population proportion of 60% is 0.6923.
Explanation:
According to the Central limit theorem, if from an unknown population large samples of sizes n > 30, are selected and the sample proportion for each sample is computed then the sampling distribution of sample proportion follows a Normal distribution.
The mean of this sampling distribution of sample proportion is:

The standard deviation of this sampling distribution of sample proportion is:
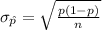
The information provided is:
p = 0.60
n = 100
As n = 100 > 30, the central limit theorem can be applied to approximate the sampling distribution of sample proportions.
The distribution of sample proportion is
 .
.
Compute the probability that in a random sample of 100 CSU graduates the error is within 5% of the population proportion of 60% as follows:
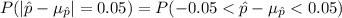
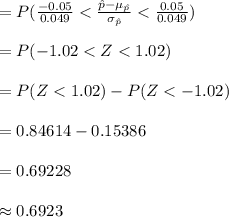
Thus, the probability that in a random sample of 100 CSU graduates the error is within 5% of the population proportion of 60% is 0.6923.