Answer:
The correct statement is (d).
Explanation:
The (1 - α)% confidence interval for the population proportion p is:
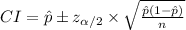
The 90% confidence interval for the proportion of all orders that arrive on time is:
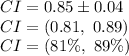
The (1 - α)% confidence interval for population parameter implies that there is a (1 - α) probability that the true value of the parameter is included in the interval.
Or, the (1 - α)% confidence interval for the parameter implies that there is (1 - α)% confidence or certainty that the true parameter value is contained in the interval.
The 90% confidence interval for the proportion of all orders that arrive on time, (81%, 89%), implies that there there is 0.90 probability that 81% to 89% of orders arrive on time.
Or, there is a 90% confidence that 81% to 89% of orders arrive on time.
Thus, the correct statement is (d).