Question

Help please! i need to find the restrictions to this expression

(x+4)(x-7)
————-
(x-7)(5x+1)

Answer 1

x = 7, x =
`(-1)/(5)`

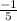
Explanation:

A restriction can only be found in the denominators and this is when x is a certain number that when plugged in, makes the expression 0 (this makes it undefined, which you cannot have).

Since we are only looking at the denominator, you can just ignore the numerator:

(x - 7) (5x + 1)

To find the restrictions, you must set them equal to 0:

x - 7 = 0

5x + 1 = 0

Then, solve:

x - 7 = 0 5x + 1 = 0

+ 7 + 7 - 1 - 1

________ ________

x = 7 5x = -1

x =
`(-1)/(5)`

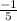
If you plug these numbers in, the total outcome would come out to be 0, making the expression undefined, proving that these are the restrictions of the expression.