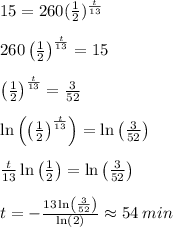Question

Element X decays radioactively with a half life of 13 minutes. If there are 260 grams of Element X, how long, to the nearest tenth of a minute, would it take the element to decay to 15 grams?

y=a(.5)^t/h

​

Answer 1

It would take 54 minutes to the element X to decay to 15 grams.

Explanation:

A radioactive half-life refers to the amount of time it takes for half of the original isotope to decay and its given by

`N(t)=N_0((1)/(2))^(t)/(t_(1/2))`

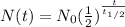
where,

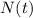`N(t)` = quantity of the substance remaining

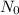`N_0` = initial quantity of the substance

`t` = time elapsed

`t_(1/2)` = half life of the substance

We know that the element X decays radioactively with a half life of 13 minutes (
`t_(1/2)`), there are 260 grams of it (
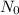`N_0`) and we want to find how long (
`t`) would it take the element to decay to 15 grams (
`N(t)`).

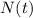Using the above formula and solving for
`t`, we get that

`15=260((1)/(2))^(t)/(13)\\\\260\left((1)/(2)\right)^{(t)/(13)}=15\\\\\left((1)/(2)\right)^{(t)/(13)}=(3)/(52)\\\\\ln \left(\left((1)/(2)\right)^{(t)/(13)}\right)=\ln \left((3)/(52)\right)\\\\(t)/(13)\ln \left((1)/(2)\right)=\ln \left((3)/(52)\right)\\\\t=-(13\ln \left((3)/(52)\right))/(\ln \left(2\right)) \approx 54 \:min`