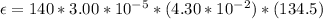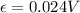Question

A generator can be made using the component of Earth’s magnetic field that is parallel to Earth’s surface. A 140-turn square wire coil with an area of 4.30 ×10−2 m2 is mounted on a shaft so that the cross-sectional area of the coil is perpendicular to the ground. The shaft then rotates with a frequency of 21.4 Hz. The horizontal component of the Earth’s magnetic field at the location of the loop is 3.00 ×10−5 T.

Calculate the maximum emf induced in the coil by Earth's magnetic field.

Answer 1

The maximum induced emf is
`\epsilon = 0.024 V`

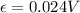
Step-by-step explanation:

From the question we are told that

The number of turns is
`N = 140 \ turns`

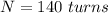
The area of the square wire coil is
`A = 4.30 * 10^(-2)m^2`

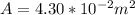
The frequency of rotation is
`f = 21.4 Hz`

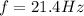
The earth magnetic field at that location is
`B = 3.00 *10^(-5) T`

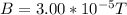
The induced emf is mathematically represented as

`\epsilon = N \ B \ A \ w sin(wt)`

At the maximum

`sin (wt) = 1`

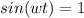
So

`\epsilon = N \ B \ A \ w`

Where

`w` is the angular velocity which is mathematically represented as

`w =2 \pi f`

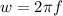
Substituting values

`w = 2 * 3.142 * 21.4`

`w = 134.5 rad/sec`

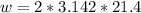
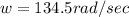
The maximum induced emf is

`\epsilon = 140 * 3.00*10^(-5) * (4.30 *10^(-2)) * (134.5)`

`\epsilon = 0.024 V`