Question

A national survey of 2000 adult citizens of a nation found that 24​% dreaded​ Valentine's Day. The margin of error for the survey was 8.9 percentage points with 85​% confidence. Explain what this means. Which statement below is the best​ explanation? A. There is 85​% confidence that the proportion of the adult citizens of the nation that dreaded​ Valentine's Day is between 0.151 and 0.329. B. There is 76.1​% to 93.9​% confidence that 24​% of the adult citizens of the nation dreaded​ Valentine's Day. C. In 85​% of samples of adult citizens of the​ nation, the proportion that dreaded​ Valentine's Day is between 0.151 and 0.329. D. There is 85​% confidence that 24​% of the adult citizens of the nation dreaded​ Valentine's Day.

Answer 1

The correct option is (A).

Explanation:

The (1 - α)% confidence interval for the population proportion is:

`CI=\hat p \pm MOE`

The information provided is:

`\hat p` = 0.24

MOE = 0.089

The 85% confidence interval for the population proportion of adults who dreaded​ Valentine's Day is:

`CI=\hat p \pm MOE`

`=0.24\pm 0.089\\=(0.151, 0.329)`

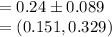
So, the 85% confidence interval for the population proportion of adults who dreaded​ Valentine's Day is (0.151, 0.329).

The (1 - α)% confidence interval for population parameter implies that there is a (1 - α) probability that the true value of the parameter is included in the interval.

Or, the (1 - α)% confidence interval for the parameter implies that there is (1 - α)% confidence or certainty that the true parameter value is contained in the interval.

So, the 85% confidence interval for the population proportion, (0.151, 0.329), implies that there is 85% confidence that the proportion of the adult citizens of the nation that dreaded​ Valentine's Day is between 0.151 and 0.329.

Or there is 0.85 probability that the true proportion of the adult citizens of the nation that dreaded​ Valentine's Day is between 0.151 and 0.329.

Thus, the correct option is (A).