Question

Suppose that the number of bacteria in a certain population increases according to a continuous exponential growth model, with a growth rate parameter of 17% per hour. Suppose also that a sample culture of 1600 is obtained from this population. Find the size of the sample after four hours. Round your awnser to the nearest integer

Answer 1

The size of the sample after four hours is of 3158 bacteria.

Explanation:

The population after t hours can be modeled by the continuous exponential growth model, which is:

`P(t) = P(0)e^(rt)`

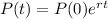
In which P(0) is the initial population and r is the growth rate paremeter.

A growth rate parameter of 17% per hour.

This means that
`r = 0.17`

Suppose also that a sample culture of 1600 is obtained from this population.

This means that
`P(0) = 1600`

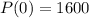
Find the size of the sample after four hours.

This is P(4).

`P(t) = P(0)e^(rt)`

`P(t) = 1600e^(0.17t)`

`P(4) = 1600e^(0.17*4)`

`P(4) = 3158.2`

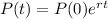
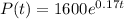
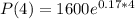
Rounding to the nearest integer

The size of the sample after four hours is of 3158 bacteria.