How does the volume of a sphere compare to that of the volume of a cylinder? What would you say the scale factor for Vol sphere:Vol cylinder

Question

asked Dec 9, 2021 122k views

1 Answer

Shahar Galukman · Answer 1 · 2021-12-15T03:46:13+0000

Answer:

The volume of a sphere is 2/3 times the volume of a similar cylinder

Scale factor : 2/3

Explanation:

Let us consider a sphere of radius r. The volume of the sphere will be given as

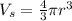
$V_(s)=(4)/(3) \pi r^(3)$

Similarly, let us consider a cylinder with its height being twice the radius of the sphere. We will have its volume given as:

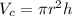
$V_(c)=\pi r^(2)h$

but h = 2r

Hence we have

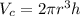
$V_(c)= 2\pi r^(3)h$

We can divide the two volumes to get a constant that links them together

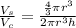
$(V_(s))/(V_(c))=((4)/(3) \pi r^(3))/(2\pi r^(3)h)$

this will give us 2/3

Hence the scale factor for Vol sphere: Vol cylinder is 2/3