Answer:
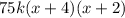
Explanation:
The first expression is
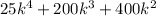
First, we extract the greater common factor
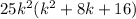
Second, we have the trinomial
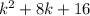
Notice that we need to find two number which product is 16 and which sum is 8, those numbers are 4 and 4.
So, the factors of the expression are
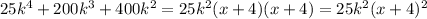
The second expression is
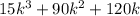
We use the same process.
Extract the greatest common factor
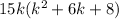
We solve the trinomial
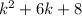 . We need to find two numbers which product is 8 and which sum is 6, those numbers are 4 and 2.
. We need to find two numbers which product is 8 and which sum is 6, those numbers are 4 and 2.
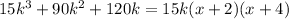
Now, the least common multiple is formed by factors that repeats in both expression.
Therefore, the least common multiple is
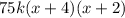 , because the LCM between 25 and 15 is 75.
, because the LCM between 25 and 15 is 75.