Answer:

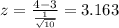
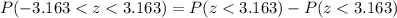
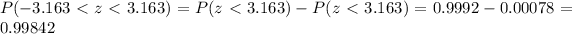
So then we will expect 9.98 packages between 2-4 rookie cards in the sample of 10
Explanation:
Let X the random variable that represent the number of rookie cards of a population, and for this case we know the distribution for X is given by:
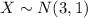
Where
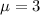 and
and
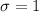
We select a sample size of n = 10 variety packs and we want to find this probability:
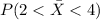
We can use the z score formula given by:
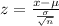
If we apply this formula to our probability we got this:
We can find the z score for 2 and 4 and we got:

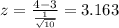
So we can find the probability with this difference
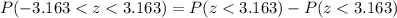
And using the normal standard distirbution or excel we got:
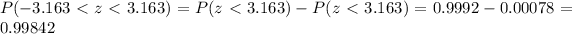
So then we will expect 9.98 packages between 2-4 rookie cards in the sample of 10