Question

Complete the 2 problems below:
Show steps

Answer 1

1). m∠ACB = 20°

2). central angle measure = 156°

inscribed angle measure = 78°

circumscribed angle measure = 24°

Explanation:

1). Measure of ∠AOB = 40°.

Since m∠ACB =
`(1)/(2)(m\angle AOB)`

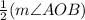
m∠ACB =
`(1)/(2)* 40`

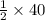
= 20°

2). Measure of the central angle of the the given circle, m ∠BOD = 156°

Inscribed angle, m∠BAD =
`(1)/(2)(m\angle BOD)=(1)/(2)* 156`

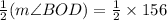
= 78°

Circumscribed angle m∠BCD =
`(1)/(2)(m\widehat{BAD}-m\widehat{BD})`

=
`(1)/(2)[(360-156)-156]`

=
`(1)/(2)(204-156)`

=
`(1)/(2)(48)`

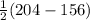
= 24°

Therefore, central angle measure = 156°

inscribed angle measure = 78°

circumscribed angle measure = 24°