Question

6 blue chips 13 pink chips 7 white chips Vicky takes out a chip from the bag randomly without looking. She replaces the chip and then takes out another chip from the bag. What is the probability that Vicky takes out a blue chip in both draws? PLEASE HELLPP

Answer 1

`\sf (9)/(169)`

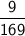
Explanation:

The bag has:

• 6 blue chips
• 13 pink chips
• 7 white chips

⇒ Total number of chips = 6 + 13 + 7 = 26

Probability Formula

`\sf Probability\:of\:an\:event\:occurring = (Number\:of\:ways\:it\:can\:occur)/(Total\:number\:of\:possible\:outcomes)`

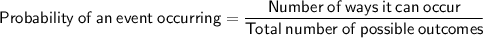
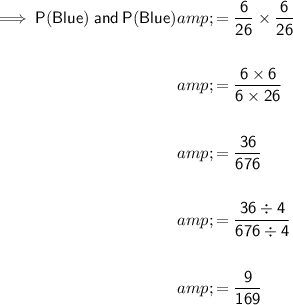
Probability of choosing a blue chip from the first draw:

`\implies \sf P(Blue)=(6)/(26)`

As the chips are replaced, the probability of choosing a blue chip from the second draw is the same as the first.

Therefore, the probability of taking out a blue chip in both draws is:

`\begin{aligned}\implies \sf P(Blue)\:and\:P(Blue) & = \sf (6)/(26) * (6)/(26)\\\\& = \sf (6 * 6)/(\26 * 26)\\\\& = \sf (36)/(676)\\\\& = \sf (36 / 4)/(676 / 4)\\\\& = \sf (9)/(169)\end{aligned}`

Answer 2

• 9/169

Explanation:

Total number of chips

• 6 + 13 + 7 = 26

Probability of a blue chip

• P(blue) = blue / total = 6 / 26 = 3/13

The subsequent blue has same probability as the chip is replaced.

Probability of two blue chips is

• P(blue, blue) = 3/13*3/13 = 9/169