Question

Use the Pythagorean theorem to find the number that will replace the ? mark,

9cm
? cm
34 cm
You are looking for the square! No units
The square is cm?

Answer 1

43

Explanation:

The sides of the squares make up the sides of the triangle.

To find the measure of a side of the square, take the area given and square root it, since the area of a square is equal to the length of its side squared.

For the 9cm^2 area, the square root of 9 is 3, so each side is 3 cm.

For the 34 cm^2 area, the square root of 34 is about 5.83, so each side is about 5.83 cm.

Plug these into the Pythagorean theorem (
`a^2+b^2=c^2`) for a and b.

You get
`9+34=c^2`.

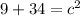Solve for c by square rooting both sides to get about 6.56.

Since it's asking for the area, square 6.56 to get 43.

An easier way to do this would be to recognize that
`a^2`,
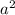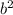`b^2`, and
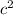`c^2` are all equal to the area of their respective squares, since the area of a square is equal to its side squared, and the Pythagorean theorem is also squaring the sides.

Using this information, a^2 is 9, b^2 is 34.

Once you add them up, you get c^2, and you know that c^2 is the area of the square the question is asking for.

Therefore, c^2 is equal to 9 + 34, or 43.