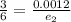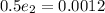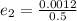Answer:
The extension of the second wire is

Step-by-step explanation:
From the question we are told that
The length of the wire is
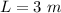
The elongation of the wire is
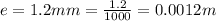
The tension is
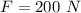
The length of the second wire is
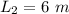
Generally the Young's modulus(Y) of this material is
Where
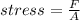
Where A is the area which is evaluated as
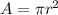
and
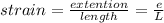
So

Since the wire are of the same material Young's modulus(Y) is constant
So we have
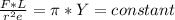
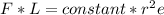
Now the ration between the first and the second wire is
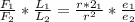
Since tension , radius are constant
We have
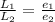
substituting values