Answer:
At least 1022 new moms should be recruited.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.
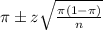
In which
z is the zscore that has a pvalue of
 .
.
The margin of error is:
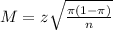
95% confidence level
So
 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
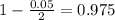 , so
, so
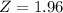 .
.
How many individuals should the researcher recruit to participate in the study if the researcher wants to be 95% confident that the margin of error is no more than 3.0%?
At least n new moms should be recruited.
n is found when
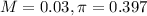
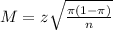
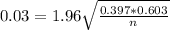
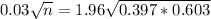
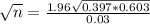
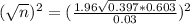

Rounding up
At least 1022 new moms should be recruited.